The Dorabella Cipher (Part 3)

Today, only a very small update regarding the Dorabella cipher.


Below you see the timeline that contains the 4 points in time at which E. Elgar made use of his cipher symbols. I am aware of four points, if someone knows another different point and can tell what Elgar did with his symbols or what he wrote about them, please notify me about that.


Timeline:

1 - - - - - - - - - - 2 3 - - - - - - - - - - - - - - - - - - - - - - 4

1: 1885/86 - That is the Lisz-Fragment; the annotation he wrote on the side of some musical nodes [Figure 5,6, in Part 1].

2: 1896 - The Courage card set. He wrote his cipher symbols [Figure 3, in Part 2] on the first card of the set of nine cards on which he explained his solution of the Pall Mall Magazin cipher challenge.

3: 1897 - The Dorabella cipher [Figure 1, in Part 1].

4: 1920 - His notebook, that contains the example subsitutions [Figure 2, in Part 1].

Based on this timeline, one can see, that the timespan between $3$ and $4$ is $23$ years. Thus the cipher scheme approach written in his notebook could very well be some kind of effort to remind his old cipher system. The two events that are close in time are $2$ and $3$, that are the symbols from the Courage card set and the Dorabella cipher. Perhaps he used the idea of the challenge cipher (Nihilist cipher) in combination with his cipher symbols to construct the Dorabella cipher?

[The Dorabella Cipher (Part 4)]

Infinite Regular Primes Conjecture

Another unproven conjecture in number theory is the Infinite Regular Primes Conjecture (IRPC). A regular prime can be characterised in more than one way.

Definition [Regular Prime]. A prime \(p > 2\) is called regular if it does not divide the class number of the \(p\)-th cyclotomic field $\blacktriangleleft$.

An alternative characterisation is the following.

Definition [Regular Prime]. A prime \(p > 2\) is called regular if it does not divide the numerator of any Bernoulli number \(B_n\) for \(n = 2,4,6,...,p-3\) $\blacktriangleleft$.

Then the IRPC conjecture simply this:

[Infinite Regular Primes Conjecture] There are infinite many regular prime.

Kummer proved in 1850 that Fermat's Last Theorem is true if the involved exponent $p$ is regular.

If there are regular primes, it is not hard to guess that there are also irregular primes. Their infiniteness as already been proved several decades ago (1954)  by Carlitz [1]. His proof is based on the second characterisation. It is a proof by contradiction; he assumes that there are only finite many irregular primes, which leads him to a contradiction to some proved result about Bernoulli numbers.

The Agoh-Giuga Conjecture

Number theory accommodates a lot of conjectures and a lot of them resist proof attempts since many years or even decades. Most of them have to do with prime numbers and its behaviour. 

One of those conjectures is the Agoh-Giuga Conjecture (AGC). Actually, it was Guiseppe Giuga who formulated the conjecture in 1950 and Takasi Agoh reformulated it 40-years later.

The original statement from Giuga was the following:

[Agoh-Giuga-Conjecture] The integer \(p\) is a prime number if and only if $$\sum^{p-1}_{k=1}k^{p-1} \equiv -1\pmod{p}$$

and the reformulation due to Agoh is

[Agoh-Giuga-Conjecture] The integer \(p\) is a prime number if and only if $$pB_{p-1} \equiv -1\pmod{p}$$

It is not hard to show that these two formulations are actually equal.

D'Agapeyeff Cipher (Part 1)

The 1939 edition of D'Agapeyeff's book

Alexander D'Agapeyeff was a russian-born cartograph living most of his time in London. He became famous for his excercise cipher, he left for the readers on the last page of the first edition of his book "Codes and Ciphers" published in 1939.

In the later revisions of his book, he removed this exercise. The reason, he said, was that he forgot how he did the encryption.

Maybe he did not really forgot how he did, but he made some mistakes that prevents him and also everyone else to decrypt the message.

What makes me a little bit upset is, that he did not reveal what he still reminded. He probably still knew what method he supposed to have used and some of the words that were contained in the message. Just as he knew that a lot of people are working on a solution, why did he not support them with a little bit of information.

The D'Agapeyeff cipher is one of those ciphers, from which is believed that it is no hoax, but a serious challenge to the cryptanalysists. Since Alexander was not a cryptologist, it is believed that he used a cipher method from his book or perhaps a combination. The cipher was also mentioned in various journal publications, like the Cryptologica [1] or the Cryptogram [2].

D'Agapeyeff cipher:

      75628 28591 62916 48164 91748 58464 74748 28483 81638 18174
      74826 26475 83828 49175 74658 37575 75936 36565 81638 17585
      75756 46282 92857 46382 75748 38165 81848 56485 64858 56382
      72628 36281 81728 16463 75828 16483 63828 58163 63630 47481
      91918 46385 84656 48565 62946 26285 91859 17491 72756 46575
      71658 36264 74818 28462 82649 18193 65626 48484 91838 57491
      81657 27483 83858 28364 62726 26562 83759 27263 82827 27283
      82858 47582 81837 28462 82837 58164 75748 58162 92000
 

Characterising integer tuples by co-prime nearby integers (Part 1)

The Chinese Remainder Theorem is a beautiful tool if someone wants to characterise a large integer \(N\) via a set \(\mathcal{B} = \{n_1,...,n_m\}\) of small integers. Based in the remainders \(\{N\pmod{n_1},...,N\pmod{n_2}\} = \{r_1,...,r_m\}\) one can reconstruct the unique integer \(N\pmod{\prod^m_{i=1}n_i}\), which is \(N\) itself if \(N \leq \prod^m_{i=1}n_i\).

What i want to discuss in this post is, if it is possible to characterise a tuple of integers \(N_1,N_2\) via their co-prime neighbors. For example:

Suppose we have \(N_1\) and \(N_2\), which are two unknown integers. All it is known, is that
$$\gcd(N_1+s_i,N_2+t_i) = 1$$
for integers \(s_i\) and \(t_i\), \(i \leq k\). These \(s_i\) and \(t_i\) could be small, i.e., 2, 3 or 5 but also any other integer. The question is:

Given the set \(\mathcal{S} = \{(s_1,t_1),...,(s_k,t_k)\}\), is it possible to say anything non trivial about the integers \(N_1\) or \(N_2\)?

If this would be possible, one could, e.g., use this information to help to factorize integers. The relationship is the following:

Lemma 1. Let \(n = pq\) and \(p,q \in \mathbb{P}\) and let \(p = gm_1+d_1\) and \(q = gm_2+d_2\) with \(\gcd(m_1,m_2) = 1\). Let \(n-d_1d_2 = t\). If \( t > \sqrt{n}\) and \(t \in \mathbb{P}\) than \(g = 1\), hence \(p-d_1\) and \(q-d_2\) are co-prime integers.

The Dorabella Cipher (Part 2)

Elgar seems to have been so familiar with his cipherbet, that he even was able to quickly write some notes or annotations. Which means, that he either learned this cipherbet by heart or he could quickly derivate the corresponding ciphersymbol from a given letter. And if so, why shouldn't it be possible that he quickly writes to Penny the more or less irrelevant note

  "P.S. Now drocp beige weeds set in it – bure idiocy – one endtire bed! Luigi Ccibunud lu'ngly tuned liuto studo two."

or reinterpreted 

"P.S. Now droop beige weeds set in it - pure idiocy - one entire bed! Luigi Ccibunud luv'ngly tuned liuto studo two."

as Tim Roberts solution suggests? In Figure 1 one can the three Pigpen circles for his solution.

Figure 1. The Pigpen circles for T. Roberts solution.

The red marked letters indicate symbols that are not used in the Dorabella cipher. Of course, he did not use this representation but used the key setence "LADY PENNY, WRITING IN CODE IS SUCH BUSY WORK", but this is how it would look like.

Figure 2. The cipherbet of Roberts (borrowed from www.ciphermysteries.com)

Applying this cipherbet to the Liszt-Fragement yields gibberish, which is not surprisingly, since he seems to (if Roberts is right) have choosen a special version for this Dorabella cipher.

To be honest, the chances that Robert's recovered plaintext is wrong is nearly negligible. If a simple monoalphabetic substitution of a ciphertext yields not only english words but also in a meaningful order, than it is hardly to believe that to be just accidential. There are so many letter-dependencies within in the text, that it this has to be correct somehow.

So, albeit i think it is overall the correct solution, it still bothers me that there are some shortcomings in its explanation.

In 1896, the Pall Mall Magazine published a code challenge, said to be "uncrackable", which finally was solved by E. Elgar. It was the Nihilist cipher and he was so proud of his solution that he painted it later on a wooden floor. He explained the solution on a set of nine cards (the Courage card set). On the first of these cards he drew the symbols:

Figure 3. The symbols from the Courage card set. Order unknown.

I am not sure if the order is correct. Since this is a full rotation of the 3-cusps symbols concatenated with the two other symbols in upright direction, its hard to believe that this encodes a word. What could have be his intention to draw this symbols on that card set?

The Dorabella Cipher (Part 1)

Below, in Figure 1, you can see the famous Dorabella Cipher, which Edward Elgar wrote to Miss Dora Penny 1897.

Figure 1. The Dorabella Cipher (1897)

You can read more details about the background of Elgar, Penny and their families in the linked wikipedia article.

What makes this cipher so special is, that it was send from Elgar (who liked to play around with ciphers and word puzzles) to a Lady, which did not have any particular background or interest in ciphers. They only met a few times and still Elgar was sure that she could somehow decipher his message.

During the years there were several proposed solutions for the cipher, but none of them got accepted by the community as being correct. It is assumed that it is a simple monoalphabetic substitution cipher and it has not been solved because of the short ciphertext, which neglects attacks based on letter frequencies.

So far, there is only one solution which seems somehow promising to me (Tim S. Roberts, Solution). It is because of the very meaningful recovered plaintext, but it still contains some really strange inconsistencies and doubtful steps. There are many websites that discussed his solution and i will not restate any of them here.

The symbols used in the Dorabella Cipher reminds one of a Pigpen Cipher. And this theory could be supported with some further hints:

The E-like symbols from the Dorabella Cipher also appear in some of Elgar's notebooks, see for example Figure 2. You can see, that Elgar is playing around in order to find a suitable letter-to-symbol configuration. On the bottom left you can see circles with a cross in it and those little marks at eight distinct positions.

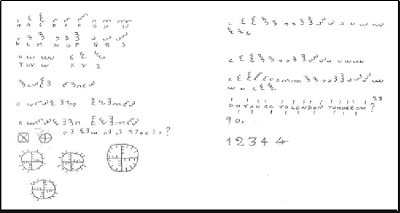Figure 2. Notes from Elgar (from 1920?) where he plays around with the same symbols.

This looks very much like a Pigpen Cipher approach to arrange symbols in a certain way to find a easy to recognize mapping from the alphabet to the cipher symbols. He also used the symbol-to-alphabet mapping, that is shown in the top left, to encode three messages on the page:

1. "MARCO ELGAR" (the name of his dog)
2. "A VERY OLD CYPHER"
3. "DO YOU GO TO LONDON"

In the 3. message, some symbols seem to be quite wrong or at least ambiguous, if we assume that he really wants to encode "DO YOU GO TO LONDON" as written in plaintext on the right side. And I have no clue why he adds the word "TOMORROW" on the right. Note that these notebook entries are made 23 years after the Dorabella cipher. Perhaps he was trying to remember his method.

Elgar used three different types of cipher symbols and each one could be oriented in eight different directions, thus in total 24 possible symbols. As usual, see also Figure 2, the letters I and J as well as U and V are combined to reduce the alphabet from 26 to 24 characters.

Figure 3. A Pigpen circle and a possible symbol mapping.

For such a Pigpen circle, there are \(2^8\) ways to orient the little marks on that \(8\) segments, either on the left or on the right side of the corresponding line. In Figure 3, you can see a possible configuration of the Pigpen circle. Since Elgar drew such circles several times, it is possible that he also used such circles for the Dorabella Cipher.

But even if he used this circles as the base for his Pigpen Cipher, in what order did he assign the letters to the circles segments? And did he used the same Pigpen circle for all 24 symbols or do they differ for the three different symbol types? Or did he somehow encoded all 24 symbols in one such circle? The last question is backed-up by the fact, that there are exactly 24 little lines on that circle. So each such line could represent a symbol rather than three of them.

This approach was also taken by Tony Gaffney and produced the, so called, Hellcat Solution.

Figure 4. Tony Gaffney's Pigpen circle for his solution.

The outcome is the text:

"B Hellcat ie a war using effin henshells! Why your antiquarian net diminuendo? Am sorry you theo o’ tis god then me so la deo da — aye"

I can not believe that this is the correct message, although i like the approach using this particular Pigpen circle.

Surprisingly, in 1885, already a decade before writing the Dorabella cipher, Elgar used these symbols to make an annotation against a couple of lines of music.

Figure 5. Elgar's annotation from 1885.

You have to rotate the page about 90 degrees clockwise in order to read the cipher symbols correctly as done in Figure 6.

Figure 6. Rotated annotation text. Known as the "Liszt Fragment".

The Pigpen circle that Elgar used for this ciphertext is nowhere shown. This fragment became known under the name Liszt Fragment. Tony Gaffney suggest for this one to use a similar Pigpen circle as for his Hellcat solution, that produces the plaintext:

"Mes it's one Frn seezhup"

Other annotations or pieces of text along the music lines in his notebook are said to be something like "Very good performance", "slightly out of tune", "poor", "beautiful", "I think you know this a little", "very well done august" and so on. Why should he wrote the itself rather cryptic sentence "Mes it's one Frn seezhup"? And why would he encrypt an musical annotation at all while leaving many others in plaintext?